Drawing a fractal tree in Python, not sure how to proceed

Question

I have this so far in python

import turtle
import math
t = turtle.Turtle()
t.shape("turtle")
t.lt(90)

lv = 11
l  = 100
s  = 17

t.penup()
t.bk(l)
t.pendown()
t.fd(l)

def draw_tree(l, level):
    l = 3.0/4.0*l
    t.lt(s)
    t.fd(l)
    level +=1
    if level<lv:
        draw_tree(l, level)

    t.bk(l)
    t.rt(2*s)
    t.fd(l)
    if level<=lv:
        draw_tree(l, level)
    t.bk(l)
    t.lt(s)
    level -=1

t.speed(100)        
draw_tree(l, 2)

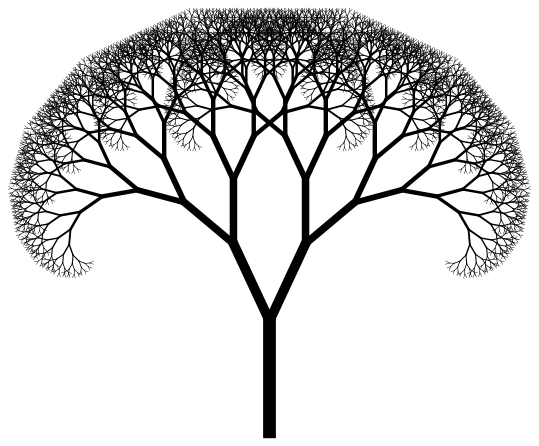
But I'm kind of stuck on how to proges, because I need to reach for building this tree. This is what I'm trying to produce:

Can any one tell me what I am doing wrong?

Answer 1

Here's HOW-TO write it in 17 lines(no functions):

import turtle
for i in range(2048): #how many branches(2 ** depth)
turtle.goto(0,0) #beginning of tree
turtle.setheading(90) #direction(try 270 for root effect)
turtle.hideturtle() #MUCH faster drawing and better view
a = str(bin(i)).replace('0b','') #binary is for two branches('0b' ignored)
a = list(str('0' * (11 - len(list(a)))) + a) #cake filling
turtle.speed(0) #no animation
turtle.pendown() #you probably wanna draw it
for f in range(len(a)): #depth
    b = 200 #primary branch length
    for l in range(f + 1): #more depth less length
        b /= 1.5 #the difference of branch length(b = b / float)
    turtle.width(b / 10) #branch width(for better effect)
    turtle.forward(b) #drawing a branch
    turtle.right((int(a[f]) - 0.5) * 60) #change 60 to any angle
turtle.penup() #also try without this line

Answer 2

I really liked @cdlane answer, so I played with the code for a while. The tree now looks much better, and the code is much more readable, so I think it is worth sharing.

The code:

import turtle

WIDTH = 15
BRANCH_LENGTH = 120
ROTATION_LENGTH = 27


class Tree_Fractal(turtle.Turtle):
    def __init__(self, level):
        super(Tree_Fractal, self).__init__()
        self.level = level
        self.hideturtle()
        self.speed('fastest')
        self.left(90)
        self.width(WIDTH)
        self.penup()
        self.back(BRANCH_LENGTH * 1.5)
        self.pendown()
        self.forward(BRANCH_LENGTH)
        self.draw_tree(BRANCH_LENGTH, level)

    def draw_tree(self, branch_length, level):
        width = self.width()
        self.width(width * 3. / 4.)
        branch_length *= 3. / 4.
        self.left(ROTATION_LENGTH)
        self.forward(branch_length)

        if level > 0:
            self.draw_tree(branch_length, level - 1)
        self.back(branch_length)
        self.right(2 * ROTATION_LENGTH)
        self.forward(branch_length)

        if level > 0:
            self.draw_tree(branch_length, level - 1)
        self.back(branch_length)
        self.left(ROTATION_LENGTH)

        self.width(width)


if __name__ == '__main__':
    tree_level = 11  # choose
    tree = Tree_Fractal(tree_level)
    turtle.done()

Answer 3

Your code is basically correct, you mostly need to adjust your parameters. The example tree you're trying to match is larger than what you are drawing (likely reduced in that image) so increase your l parameter. The example tree has a couple more levels of recursion than yours so increase your lv parameter.

Finally, you need to reset the pen width based on the recursion level (and unset it on your way out.) The following rework of your code does this but needs further fine tuning:

import turtle

t = turtle.Turtle(shape="turtle")

t.lt(90)

lv = 13
l = 120
s = 17

t.width(lv)

t.penup()
t.bk(l)
t.pendown()
t.fd(l)

def draw_tree(l, level):
    width = t.width()  # save the current pen width

    t.width(width * 3.0 / 4.0)  # narrow the pen width

    l = 3.0 / 4.0 * l

    t.lt(s)
    t.fd(l)

    if level < lv:
        draw_tree(l, level + 1)
    t.bk(l)
    t.rt(2 * s)
    t.fd(l)

    if level < lv:
        draw_tree(l, level + 1)
    t.bk(l)
    t.lt(s)

    t.width(width)  # restore the previous pen width

t.speed("fastest")

draw_tree(l, 2)

turtle.done()